The following games are thrown out when using Solitaire Games of Skill program except in the case when a solver shows the game is unwinnable. In that case the player will have to opt out of the game himself. Please note that in general, unless you are using a solver, not all the unwinnable games will be thrown out.

• When less than four cards of a given rank are available for pairing when playing Pyramid
  Two cards whose ranks add to 13 can be paired and removed when playing Pyramid, the object being to remove all the cards. Thus a card of rank ten can be paired with a card of rank three. The screenshot below shows four cards of rank three in the square covering a card of rank ten in the circle. The three tens not covered by the threes could be used to remove three of the four threes in the square. But one three would still be left covering the ten in the circle. Therefore the game would be unwinnable since the only card which could be used to remove the three is the ten covered and made inaccessible by the three. Conversely if one card of rank ten covered all four rank three cards, the game would be unwinnable since there would be no card available which could be paired and removed with the covering ten. Even if two cards of rank ten cover three cards of rank three in the Pyramid, the game might be unwinnable. There are many other similar scenarios which would make a game unwinnable.
  
  



• When a space in the tableau cannot be made
  If you cannot make a space in the tableau when playing Fortress, Streets and Alleys, Beleaguered Castle, Citadel, Flower Garden, and King Albert the game is lost. Sometimes this can be seen at the start of the game. Other times you have already wasted a considerable amount of your time before you discover the hopelessness of the situation. In the screenshot of a Fortress game below, only the ace of spades in column 2 can be moved.
  
  



• When facedown cards cannot be uncovered
  When playing Scorpion, Russian Solitaire, or Alaska if the faceup card covering a column of facedown cards cannot be moved the game is lost. When playing Scorpion and Russian Solitaire tableau cards of the same suit can be built down on each other. In Scorpion there is no foundation. If when playing Scorpion a faceup nine of spades covers a column of facedown cards which includes the ten of spades, the nine of spades can never be built on the inaccessible ten of spades. Therefore the facedown cards covered by the nine of spades can never be uncovered, making the game unwinnable. If the above situation occurs in Russian Solitiare, the game would be lost if in addition the nine of spades covers a card of the same suit and lower rank. In this case the nine of spades could not be moved to the foundations, making the game unwinnable. When playing Alaska tableau cards of the same suit can be built up or down on each other. Thus the game of Alaska would be unwinnable if a faceup nine of spades covered facedown cards which included the eight and ten of spades.



• When a group of three consecutive ranks occurs with the smallest rank in the middle
  If three consecutive cards of the same suit such as the nine, eight, and seven of clubs occur together with the smallest rank in between and the largest rank first, the game is lost when playing Bisley, Scorpion, Russian Solitaire, and Australian Patience. In these games tableau cards of the same suit can be built down on each other. Notice the 9 - 7 - 8 of clubs sequence circled in the Scorpion screenshot below. The only card which could move the seven of clubs is the eight of clubs which is directly below it and thus is incapable of moving the seven of clubs. Therefore there is no way to get at the seven of clubs. The game is unwinnable.
  
  



• When two cards are covered by each others card one rank below in suit sequence
  Tableau cards of the same suit can be built down on each other when playing Scorpion. In the Scorpion screenshot below the jack of clubs is covered by the jack of spades in column 3 while the queen of spades is covered by the ten of clubs in column 5. In order to build the jack of spades on the queen of spades, the ten of clubs would have to be moved. But in order for the ten of clubs to be moved to the jack of spades, the jack of clubs would have to be moved. Thus the jacks in column 3 cannot be split apart rendering the game unwinnable. Scorpion is the only game where this kind of block can occur.
  
  



• When two cards are covered by each others card two ranks below or above in suit sequence
  Tableau cards of the same suit can be built on each other either up or down when playing Bisley. In the Bisley screenshot below the six of hearts is covered by the eight of hearts in column 5 while the nine of hearts is covered by the seven of hearts in column 6. In order to build the eight of hearts on the nine of hearts the seven of hearts would have to be moved. But in order for the seven of hearts to be moved to the six of hearts the eight of hearts would have to be moved. Thus the pairs of hearts in columns 5 and 6 cannot be split apart by tableau building. Also none of these cards can be sent to the foundations since the six of hearts and nine of hearts are inaccessible. The game is unwinnable. In addition to Bisley this kind of block can occur in Fan and La Belle Lucie.
  
  



• When all the cards in a stock cannot be uncovered
  When playing Canfield or Chameleon if all the cards in the stock cannot be uncovered, the game is lost. This is the case if a stock card covers all the cards with which it can be built on the tableau and any card needed to build it on the foundations. In the game Canfield cards can be built downward on the tableau in alternating color. Let us say that while playing Canfield the ten of hearts is a stock card and below the ten of hearts in the stock are the two black jacks. In this situation the ten of hearts could never be moved off the stock and built on a black jack on the tableau since the black jacks are inaccessible. Furthermore if below the ten of hearts in the stock is a card of the same suit and lower in the foundation sequence, the ten of hearts could never be moved to the foundations. Thus the game would be unwinnable.



• When a card covers a card of the same suit and one rank above and a card of the same suit and a lower rank
  Tableau cards of the same suit can be built down on each other when playing Fan, La Belle Lucie, Cruel, and Perseverance. Often when playing these games, a card covers a card of the same suit and one rank above and a card of the same suit and a lower rank. If this pattern occurs in the first tableau column of Cruel or Perseverance or anywhere in the tableau of Fan or of La Belle Lucie with no deals left, the game is unwinnable. For example in the first column of the Cruel screenshot below, the five of clubs covers the six of clubs and the three of clubs. Redealing the cards will not change this pattern. Since the six of clubs is inaccessible the five of clubs cannot be built on the tableau. Since the three of clubs is inaccessible the five of clubs cannot be built on the foundations. Therefore the game is unwinnable. If a king covers a card of the same suit in the first column of Cruel and Perseverance the game is unwinnable. If a king covers a card of the same suit anywhere in the tableau of La Belle Lucie when there are no deals remaining, the game is unwinnable.
  
  



• When kings cover cards of each others suit
  When playing Baker's Dozen, Shamrocks, La Belle Lucie, and Intelligence if kings cover cards of each others suit the game can be unwinnable. The foundations in these games start with aces which are built up in suit to kings. In the Shamrocks screenshot below, the king of diamonds covers the five and nine of hearts in column 5. Therefore the king of hearts cannot be removed to the foundations until the king of diamonds is moved to the foundations. The king of diamonds blocks the king of hearts. But in similar fashion the king of spades in column 13 blocks the king of diamonds. Thus both the king of diamonds and the king of spades block the king of hearts. In column 9 the king of clubs covering spade cards blocks the king of spades. Thus the kings of diamonds, spades, and clubs all block the king of hearts. But in column 12 the king of hearts covers the two of clubs. Thus the king of hearts blocks the king of clubs which in turn blocks the king of hearts. Therefore the game is unwinnable. This kind of pattern which makes winning impossible can involve two or more kings. For the game Intelligence this kind of block would only be an issue during the last redeal.
  
  


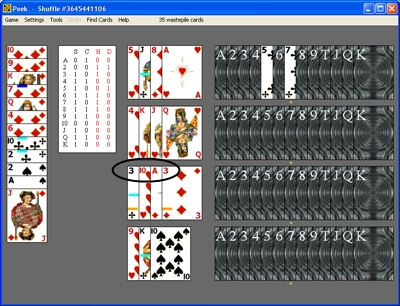
When a card covers the card it must be built upon on the foundations
All the play consists of moving cards to the foundations when playing Osmosis and Peek. The object is to move all the cards to four rows with all the cards of a given row having the same suit. For the foundations there is an index rank. The first card built on each row has to have the index rank. In the upper right of the Peek screenshot below, the five and seven of clubs are on the first row of the foundations. Any card whose suit is clubs can be moved to the top foundation row. The index rank is seven. Thus any card of rank seven can be built on the second row. That determines the suit of that row. Then any other cards of the same suit can be built on that row as long as a card of the same rank is in the row above. Thus after the seven of diamonds was placed underneath the seven of clubs on row two, the five of diamonds could be placed under the five of clubs. But the three of diamonds could not be placed because there would not be a three of clubs above it on the foundations. Inside the circle of the screenshot the three of diamonds is shown covering the three of clubs in the stockpiles. Since the three of clubs is inaccessible, the three of diamonds can never be moved to the foundations, making the game unwinnable. Also before the three of diamonds could be moved to the foundations, the seven of diamonds must be built there first. If the three of diamonds covered the seven of diamonds in the stockpiles, the game would be impossible to win. About half of the Osmosis and many of the Peek games are unwinnable as a result of the above two scenarios.




• When a solver indicates the game is unwinnable
  The tableau layout for FreeCell and Two Cells games is identical to the layout for Microsoft FreeCell games for a given game number. Thus you can determine if a FreeCell or Two Cells game is winnable using FreeCell Pro. FreeCell Pro can be downloaded for free from Mike Keller's website.